As far as I understand, the RG theory, or functional RG theory is a mathematical tool for moving in the "scale dimension". The tool can be used for calculation of Feigenbaums constant (e.g. mentioned here). Can the theory be given a simple example of how to move one "step" in the "scale dimension" ?
[Math] Simple example of renormalization
ds.dynamical-systemsmp.mathematical-physicsquantum-field-theoryrenormalization
Related Solutions
There a number of papers by Alain Connes on Dimensional Regularization (Dim Reg) in the context of noncommutative field theory. Some of his papers cite
P. Breitenlohner and D. Maison, "Dimensional renormalization and the action principle," Comm. Math. Phys. Vol. 52, Number 1 (1977).
so I presume this might be a useful reference for you.
Regarding your observation, this is a well known fact among particle physicists and is regarded as a feature rather than a bug. Part of the standard lore is that Dim Reg replaces log divergences by poles but that linear and higher divergences often just give zero. Physicists are most interested in log divergences since these govern violations of scale invariance, determine beta functions and so on. In your example the integral has a pole at d=2 where the integral has a log divergence but vanishes at d=3 where the divergence is linear.
There is a beautiful article (a write-up of a talk, actually), by E.M. Purcell, Life at low Reynolds number, that explains how bacteria swim.
Low Reynolds number is the technical way to phrase the statement in the OP that motion at that scale feels like moving in a tar pit. The governing equation is the linearized Navier-Stokes equation, a.k.a. the Stokes equation, which lacks the inertial $v\nabla v$ term. The linearity of the Stokes equation means that the swimming technique which we would use, moving arms or legs back and forth, will not work. Purcell calls this the "scallop theorem": opening and closing the shells of a scallop will just move the object back and forth, without net forward motion.
Inertia can still play a role on short time scales, as explained in Emergency cell swimming.
The way bacteria move in the absence of inertia is the way a corkscrew enters a material upon turning, the cork screw being the flagellum. In fact, any nonsymmetrical object, when turned will propagate in a tar pit. Typical velocities are $1$ mm/min, as Purcell says: "Motion at low Reynolds number is very majestic, slow, and regular."
Here is a visualization of a sperm cell moving by rotating its flagellum (published just this week in Science Advances).
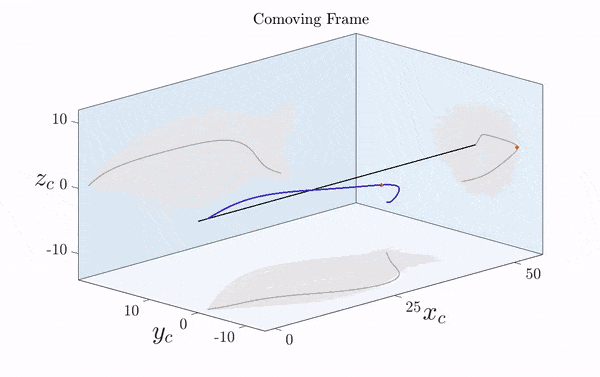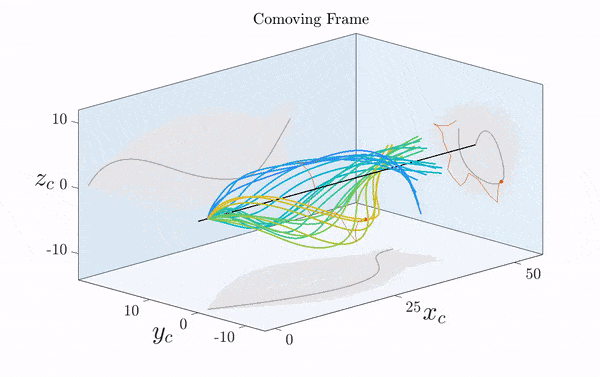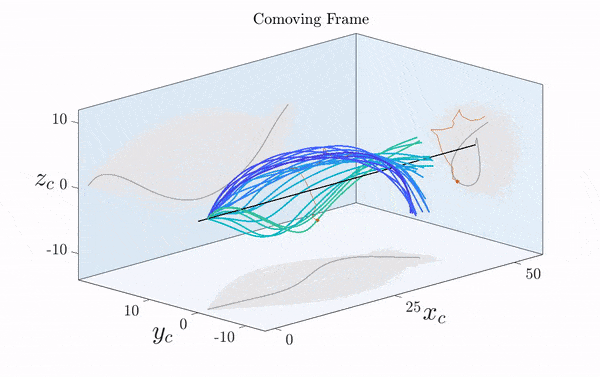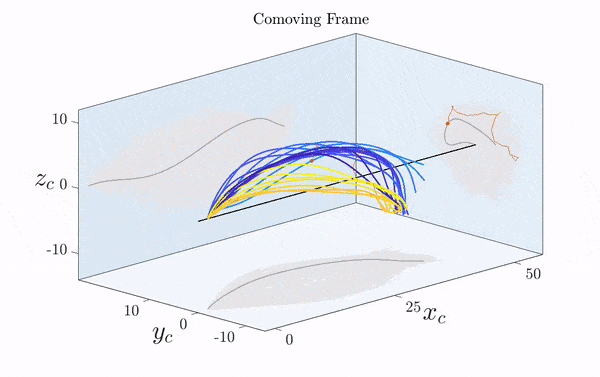
Note that the rotation is only clearly visible in three dimensions. Two-dimensional projections suggest a beating motion (first reported by Van Leeuwenhoek in the 17th century), which is not an effective means of propagation at low Reynolds number.
Best Answer
The simplest and earliest example I know regarding the renormalization group idea is the following.
Suppose we want to study some feature $\mathcal{Z}(\vec{V})$ of some object $\vec{V}$ which is in a set $\mathcal{E}$ of similar objects. Suppose that unfortunately this question is too hard. What can one do?
The renormalization group philosophy is try to find a "simplifying" transformation $RG:\mathcal{E}\rightarrow\mathcal{E}$, such that $\mathcal{Z}(RG(\vec{V}))= \mathcal{Z}(\vec{V})$, and $\lim_{n\rightarrow \infty} RG^n(\vec{V})=\vec{V}_{\ast}$ with $\mathcal{Z}(\vec{V}_{\ast})$ easy to understand.
Example (Landen-Gauss, late 1700's):
Let $\vec{V}=(a,b)\in\mathcal{E}=(0,\infty)^2$ and consider $$ \mathcal{Z}(\vec{V})=\int_{0}^{\frac{\pi}{2}} \frac{d\theta}{\sqrt{a^2 \cos^2\theta+b^2\sin^2\theta}}\ . $$
A good choice of renormalization transformation here is $RG(a,b)=\left(\frac{a+b}{2},\sqrt{ab}\right)$, as discovered by Gauss.
A recent example now.
Example (Kadanof-Wilson, late 1960's early 1970's):
Take $\mathcal{E}$ to be the set of Borel probability measures on $\mathbb{R}^{\mathbb{Z}^d}$. Let $\mathcal{Z}(\vec{V})$ be equal to $1$ if the two-point function decays exponentially and $0$ otherwise.
Then define $RG$ as the transformation which gives the law of the block-spinned/coarse-grained field as a function of the law of the original field.
Note that the feature $\mathcal{Z}$ that one would like to preserve can be defined a bit more loosely. One could, e.g., "define" it as the long-distance behavior of the random spin field with probability law $\vec{V}$ (or in physics jargon: the low energy effective theory). Also in the dynamical systems context, it could be the chaotic behavior or not of a map $\vec{V}$. Then $RG$ could be a doubling transformation, i.e., composition of the map with itself together with some rescalings and reversals of orientation.